Definition and assumptions
Discounting of cash-flows is a financial process that computes the value today of a stream that you will generate in the future. At constant value, a cash-flow projected into the future is worth less than a cash-flow today for two reasons:
1. we prefer to consume today rather than tomorrow. In order to ensure that a certain individual does not consume a certain good today, we have to grant him that in the future he will have the possibility of consuming larger, the real rate of return;
2. in calculating cash-flows that we expect will be generated in the future, we must take into account some degree of risk or uncertainty which consequently reduces the value in proportion to time estimates.
For this reason, the higher the risk, the greater the difference between a cash-flow that we think we earn today than one of the same projected balance in the future.
In addition, we may need to take into account the effect of monetary inflation: the value of the currency is not constant over time, but decreases from year to year. This means that, for the same amount between two cash-flows, the higher the inflation rate, the greater the difference between the value of a flow generated this year and one next year (and so on).
The process of discounting
In order to take account of the reasons described above, cash-flows must be “fixed” by multiplying each of them for a discount factor through the following formula:
Present value of a cash-flow =
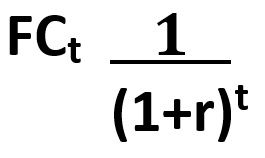
r is the discount rate
FCt is the cash-flow at time t
The value 1/(1+r)t is called the discount factor and corrects cash-flow taking into account the period in which it is determined. The higher the discount rate, the lower the present value of future cash-flows, so that present value and the discount rate are inversely proportional.
But, what is actually the discount rate r? As previously mentioned, there are two reasons why you discount cash-flows:
1. yield waived by deciding to invest in that project rather than in a financial asset with the same risk;
2. a greater degree of risk leads to a reduction of the value in the future.
The net present value of an investment project is given by the present value of the cash-flows that the project can generate over time less the value that would be obtained through alternative investments having the same degree of risk. The fair value of alternative investments enables you to locate a particular rate called cost opportunity of capital, interpretable as the rate of return expected by investors for an investment in stocks or bonds that have the same risk. Let’s take a very simple example.
Suppose you want to buy a property that today is worth $ 700,000 but in a year, after a renovation that will cost an additional $ 300,000, we are sure it will have a value of $ 1,200,000. This is not however the only safe investment I can make. We imagine that by investing in the purchase of bonds of a certain company that I guarantee 8% interest a year. In such a case to get $ 1,200,000 a year from now should I invest now $ 1,200,000/(1 + 0.08) = $ 1,111,111. This means that the present value of $ 1,200 a year, at a rate of 8% is $ 1,111,111. Let us go forward in reasoning and assume that, once you buy the property, you change your mind and decide to sell it after a month. How would pay any investor to buy? Exactly $ 1,111,111.
This applies with equal risk. The question becomes complicated if we wanted to also take into account the fact that the chosen project has a higher degree of risk than other alternatives. In this case, if for example the real estate deal was riskier, the rate of return of the bond should consider and also add a greater risk. This would result in a higher discount rate, and then a value of future cash-flows associated with that minor investment thereby promoting other less risky investments.
The consideration of inflation
As you can see, in the above formula, is not mentioned inflation that can be considered in two different approaches:
a) you can disregard it, if the analysis is made for an investment project within the EU, given that inflation is currently under control (solution we many times we privilege);
b) you can use the law of compounding: If we consider inflation as aprice increases and we call it ri, the prices will grow by factor 1 + ri for the first of the years concerned and of the factor (1+ri)t for the remaining. If the price of an asset to date amounts to p0, a year from now, because of inflation, its expected price p1 will be:
p1= p0*(1+ri)
for subsequent years (that is, over a year):
pt= p0*(1+ri)t
Similarly, the actual cash-flow calculations is obtained considering the inflation rate:
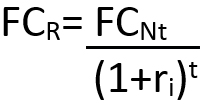
where:
FCR = actual cash-flows
FCN= nominal cash-flows
ri = inflation rate
The mistake that many times is made in the application of this method is to “forget” to apply an inflation rate even at cost items.
The choice of cash-flows to discount The cash-flows from discounting may be multiple, so here we will consider the two types most commonly used in practice and invoked in literature:
Free Cash-flow to Equity (called Levered cash-flow)
This cash-flow is what comes after considering all operational costs and taxes, the refund of net financial debt and financial charges. In other words, it is given by the resources remaining after facing the financial requirements and have paid all inputs. In practice this corresponds to, in case you decide not to pay dividends, the cash of the balance sheet.
Earnings Before Interest and Taxes (EBIT)
– Interest Expense
– Income Taxes
= Net Profit
+ Amortization
+ Provisions and Other Non-cash Items
+/- Increase (decrease) in Working Capital
+ Divestments
+/- Proceeds from Issue of Capital
+/- Increase (Decrease) in Net Financial Debt
= Free Cash-flow to Equity (FCFE)
Free Cash-flow to the Firm (also called Unlevered cash-flow)
This cash-flow, defined as “net operating” is the one generated taking into account only the operating activity of the company and its ability to remunerate equity capital and third parties. Is calculated by subtracting the net investments (Capex) to operating cash-flow but excluding repayment of debt, payment of financial charges and the remuneration of shareholders.
It is defined as: “free cash-flow for shareholders and lenders,” because the two types of lenders have not yet been paid when it is determined and its measure defines precisely the financial resources available for their remuneration. Being a value based on the company’s operating activities alone, for its proper determination you must also consider the taxes attributable to the operating result, namely the amount of taxes that the company would pay if it didn’t deduct interest expenses or extraordinary income/expenses from taxable income. Therefore, NOPAT is calculated as the difference between EBIT and these notional taxes. In this way the tax value is higher than the effective taxes.
Earnings Before Interest and Taxes (EBIT)
– Tax on operating profit (notional)
= Net Operating Profit After Taxes (NOPAT)
+ Amortization
+ Provisions and Other Non-cash Items
+/- Increase (Decrease) in Working Capital
– Investments
+ Divestments
= Free Cash-flow to the Firm (FCFF)
The choice of the discount rate to be applied to the cash-flow
The question that now we will try to answer is the following: is the discount rate to be applied unique to the two cash-flows?
The answer is that to determine the appropriateness of an investment are regarded different discount rates depending on the type of chosen stream (Levered or Unlevered):
- Free cash-flow to Equity has to be discounted at a rate that expresses the cost opportunity of comparable alternative investments (Capital Asset Pricing Model – CAPM method); in that case the rate to be used will reflect only the cost of capital corresponding to the required return by shareholders, not having considered the indebtedness;
Capital Asset Pricing Model:
Ke = rf + ß (rm – rf)
where:
rf net yield of risk-free investments;
(rm – rf ) represents the greater risk of the stock market compared to risk-free investments;
ß is the coefficient that quantifies the risk of single firm compared to market average;
- Free Cash-flow to the Firm is discounted at a rate expressing the cost opportunity of alternative investments which have an equal degree of risk of all funders (so Equity and Debt). This rate is also called the weighted average cost of capital (WACC – Weighted Average Cost of Capital).
The alternative between using the two streams considered derives both from information that we possess and from the end result we want to achieve.
The WACC is used to discount the net operating cash-flow for business valuation purposes and to calculate NPV. The WACC is the weighted average cost of all resources invested in the company, which is the weighted average of the “costs” of the different sources of funding released for company, eg. equity, bank debt, bonds, shareholder loans etc. (excluding liabilities which do not involve interests such as Payables to suppliers). This rate represents the minimum acceptable performance for the profitability of an investment required by different stakeholders who fund the company, divided into: suppliers of risk capital and debt.
WACC = kd * (1-t) * D/(E+D) + ke * E/(E+D)
kd * (1-t) = cost of debt, net of tax (interest rate paid net of tax benefit represented by the deductibility of interest expense)
D = debt value weighted by interests (mainly gross financial position)
E = value of Equity (equity)
Ke = cost of equity or risk = Rf + β * (Rm – Rf) , where:
Rf = risk-free yield or bonds in 10 years
β = systematic risk coefficient that indicates how varied the yield of a security with the variation of productivity of the entire market. This index can be found on Bloomberg, Slash, Merrill Lynch Beta Book, Value Line etc.
Rm = theoretical yield of market expected from a portfolio of risky securities
(Rm – Rf) = market risk premium.
For this reason, a project will be convenient if the performance of the project will be higher than the WACC, that means that if the NPV is positive (the two conditions are equivalent). In this case we can conclude that the investment is worthwhile simply because it offers a return higher than alternative investments of equal risk.

